Synthesis and Characterization of BiFeO3-Reduced Graphene Oxide Nano-Composites – A Potential Candidate for Future Devices
Abstract
The decrease of fossil fuels generated an intensive search for alternative natural energy sources. This also triggered research of new storage devices with high storage, power density and long cycle life. Supercapacitors whose storage capacity is much higher than electrolytic capacitors and batteries are important in this regard. In this project, a nano-composite system of BiFeO3 (BFO) and reduced graphene oxide (rGO) was synthesized using a facile hydrothermal method. Graphene oxide was synthesized by modified Hummer’s method. The ratio of rGO was varied in the nanocomposite to achieve desired results. The prepared samples have been studied X-ray diffraction method, Raman spectroscopy, and Vibrating sample magnetometer. X-ray diffraction studies confirmed the rhombohedrally distorted structure for BFO with R3c symmetry. Along with the diffraction peaks of pure BFO, low-intensity peak at 26.87o which corresponds to (002) plane of rGO with d-spacing of 0.345 nm was also observed. This confirms the successful reduction of GO. All expected Raman modes associated with BFO phase were observed in the case of pure BFO and BFO-rGO nanocomposite. Moreover, D and G Raman modes have been observed in GO and BFO-rGO nanocomposite system which are the characteristic vibrational modes of all carbon allotropes. The 𝐼D/𝐼G ratio also confirmed the successful reduction of GO into rGO in case of the composite system. Weak ferromagnetic behaviour has been observed for BFO and BFO-rGO nanocomposite. The observed magnetism has been attributed to the finite size effects in BFO where the particle size (35 nm) is less than the length (62 nm) of the spiral spin structure. A slight decrease in magnetization observed for nanocomposite is ascribed to the non-magnetic nature of rGO phase. Electrochemical properties results are awaited where we are expecting the designed nanocomposites will have improved capacitance and cyclic performance. We expect that BFO-rGO nanocomposite can be a promising candidate for high-performance super-capacitance.
1. Introduction
1.1 Introduction and background:
With the fast-growing demand for portable electronic devices such as cell phones, laptops, notebook computers,, and the development of hybrid electric vehicles, there is an urgent need for high power storage devices [1]. Several storage devices like batteries and solar cells are in our daily use and a few are being used in industrial applications such as supercapacitors (SCs) [2]. SCs are very important class storage devices that have many benefits compared to other devices. SC is also known as ultra-capacitor whose capacity is much higher than electrolytic capacitor and rechargeable batteries. . Due to the characteristics of outstanding power efficiency, excellent reversibility, very long cycle life (> 1 000 000 cycles), simple operating mode, and easy integration into electronics, SCs replace electrolytic capacitors and batteries. [3]. SCs are used in cars, buses, trains, and cranes for regenerative braking, short term energy storage, or burst mode power supply. The performance of a super-capacitive material is judged by Cyclic Voltammetry and Galvanοstatic charge-discharge measurements.
In this project, we will synthesize a BFO-RGO nanocomposite system for the electrode material of a super-capacitor. The ratio of RGO will be varied to obtain the desired results.
1.2 Principles of Conventional capacitors and Super-capacitors:
1.2.1 Principle of Conventional capacitors:
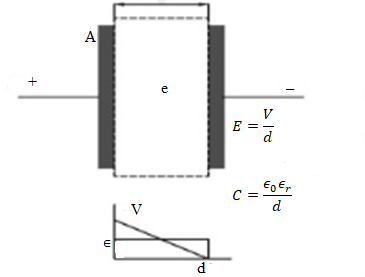
Conventional capacitors are a major source of electrical circuits used to store electrical energy in the order of microfarads. Capacitors are used for two basic purposes; one of which is to charge or discharge electricity. The function of capacitors is to charge or discharge electricity which is used for different applications such as smoothing circuits for transmission lines, microcomputer backup circuits, and timer circuitry. Another basic purpose of capacitors is to block direct current which have also several applications such as used in filters to eliminate particular frequencies.
Figure 1.1: Schematic representation of Conventional Capacitor.
The work on the technology of capacitors has been started after the invention of the Leiden jar in 1745. Now, capacitors are widely used in industrial applications such as in automobiles, space and aircraft, medicines, power supplies, games, and computers.
Capacitors consist of two conducting metallic plates which are mainly made of silicon. Both plates accumulate opposite charges separated by the dielectric medium which increases the capacity of charges in capacitors. Most used non-conducting dielectrics are glass ( ), ceramic ( ), polyethylene ( ), paper ( ), mica ( ), air ( ) and insulated mineral oil ( ). A capacitor works when a potential difference is applied across the conducting plates with the help of a battery then the electric field is developed, and this electric field is directly proportional to the potential difference and inversely related to the distance between the conducting plates which is given as.
1.1
In the given relation E is an electric field that is developed across the plates, V is potential difference and d is the separation between two metallic plates. Capacitor stores electrical energy in the form of electric field and electron flow until the potential difference between conducting plates become equal to the voltage of power supply and this is called charging of the capacitor. Now removes the battery then electron flow from the negative side toward the electron deficiency side until both plates become neutral and this is called discharging of the capacitor. The strength of the capacitor is defined by the given relation.
1.2
In this given relation C is the capacitance of the capacitor, A is the area of electrodes, d is the thickness of the dielectric, and is the dielectric constant. The unit of capacitance is Farad which is a very big unit so smaller units like , pF, and mF are used to define capacitance of the capacitor. The range of capacitance of a conventional capacitor is 0.1 to 1 with a voltage range of 50-400 V. To increase the capacitance of the capacitor, the value of dielectric constant or surface area should be increased. However, research work is in progress for the modification of conventional capacitors by increasing the specific capacitance and according to a recent report Si, and ZnO have approximately thirty times higher capacitance densities [4].
1.2.2 Principle of Super-capacitors:
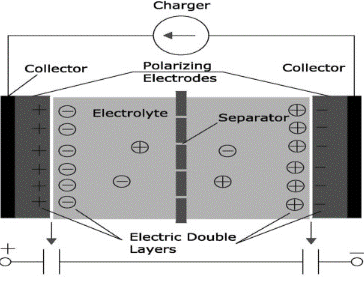
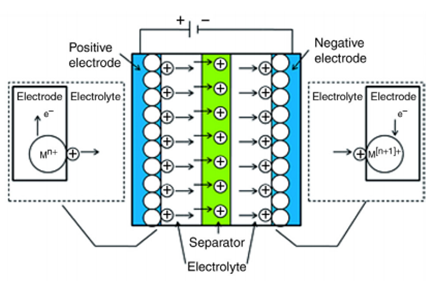
Super-capacitors are also known as Electro-chemical double-layer capacitors which store energy within an electrochemical double layer at the electrode/electrolyte interface with a higher capacitance than other capacitors but with a low voltage limit that link the gap between electrolytic capacitors and rechargeable batteries
The working principle of electrochemical capacitors is based on two electrodes where charges are accumulated, separated by an ion-permeable membrane called a separator, and ions of opposite charges are arranged on the electrolyte side (shown in figure 1.2). The total capacitance of double-layer capacitors depends on two storage principles. One is electrical double-layer capacitance and the other one is pseudo/super capacitance. The energy storage of electrical double-layer capacitors achieved at the electrode/electrolyte interface in the Helmholtz double-layer and the energy storage of pseudo/supercapacitors achieved by faradaic redox reactions. In electrochemical capacitors, the electrode material is made up of carbon while for the latter it is made up of transition metal oxides.
Figure 1.2: Configuration of typical Electro-chemical capacitor.
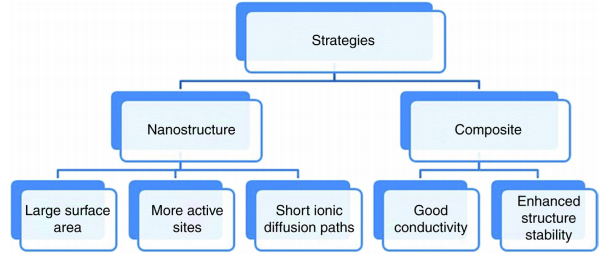
Electrode material plays an important role in the construction of super-capacitors so; to get high-performance supercapacitors the electrode material should be efficient and effective. Recently, attention has been focused on porous nanostructures as the electrode material to get high capacitance, conductivity, and efficiency.
Figure 1.3: Schemes to increase the performance of super-capacitors [5].
Electro-chemical or Super-capacitors have a high-power density of > watts per at low energy density as compared to other low-temperature fuel cells and batteries. Due to the negligibly small chemical charge transfer involved these capacitors have a very long life cycle. The key objectives of researchers working on these capacitors are the production of electric vehicle engines and the rapid growth of electronic devices that use maximum energy with high power and have the lowest possible weight and scale [6].
1.3 Types of Supercapacitors:
Electrochemical double-layer capacitors (EDLC’s), pseudocapacitors, and hybrid capacitors are categorized into super-capacitors.
1.3.1 Electrochemical Double Layer capacitors (EDLC’s):
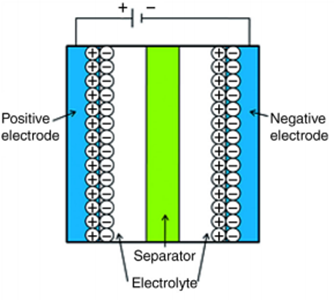
EDLC is a double-layer capacitor that accumulates charges at the electrode-electrolyte interface and is selected as a super-capacitor or ultra-capacitor. For the construction of EDLC’s carbon is used as the electrode material to get higher capacitance as output. In EDLC’s, during the charging phase, electrons pass from negative electrodes to positive electrodes through the external loop while in inverse order during the discharging phase. Whereas in the case of ions cations migrate toward a negative electrode and anions migrate toward a positive electrode during the charging phase and in reverse order during the discharging phase as shown in Fig. 1.3).
Figure 1.4: Electrochemical Double Layer Capacitors (EDLC’s).
As an electrode material, the carbon in EDLC is used to increase the capacitive double-layer charge and increase the surface area, further increasing the contact between the deposited materials and the electrolyte. The major disadvantage of using EDLCs based on carbon is lower specific stored energy. [7].
1.3.2 Pseudo-capacitors:
Capacitance is obtained by Faradaic electron charge transfer with redox reaction, electrostatic repulsion, or electro-sorption in this form of super-capacitors and occurs on electrode generating charges. These types of super-capacitors have low power density than EDLC’s due to redox reaction because Faradaic reaction is a slow process but have high specific capacitance and energy density due to Faradaic reaction (shown in figure 1.4).
Figure 1.5: Schematic diagram of Pseudo-capacitor.
For the construction of pseudo-capacitors, the electrode material is made up of metal oxides or conducting polymers which are an attractive alternative because of high capacitance at low resistance and have high energy and power. The most favorable metal oxide is which have a high capacitance of 720/900 F/g but the rarity of this oxide diverted the researchers towards other transition metal oxides [8]. Other oxides like NiO, Ni Mn , FeO, , , , , MoO have been studied as electrode material but not used for commercial production of super-capacitors. One more metal oxide considered as an electrode material for pseudo-capacitance applications is manganese dioxide due to its low cost and environmental friendliness and its capacitance reaches 1100 C/g.
1.3.3 Hybrid capacitors:
In hybrid capacitors, charges are stored by both mechanisms, i.e., electrostatically, and electrochemically because of having both characteristics of electrodes. It uses polymers to form the entire electrolyte or in conjunction with liquid electrolyte. These types of capacitors are better than electrolytic capacitors and ceramic capacitors. Their characteristics include stability, longevity, reliability, safety, and overall life cycle cost and offer a voltage range from 25 to 80 V with capacitances between 10 and 330 μF. In hybrid capacitors, the best features of EDLC’s and Lithium-ion batteries are combined while the charges are stored electrostatically in EDLC’s and electrochemically in lithium-ion batteries. This capacitor has two electrodes one is electrostatic and another one is electrochemical which gives better energy density than EDLC’s.
For the construction of a hybrid capacitor, the positive electrode is made of activated carbon which is immersed in a liquid electrolyte together with a negative electrode which is made of carbon material doped with lithium ions which are shown in Fig. 1.5 [9].
1.4 Graphene oxide (GO) as super-capacitor:
Super-capacitor has been regarded as an effective energy storage system for flexible solid-state devices and for this purpose, many flexible electrode materials have been investigated including many transition metal oxides and carbon-based materials like graphene, carbon nanotubes, and carbon nanofibres [10]. Graphene has two dimensional (2D) crystalline structures and its charge carriers, for example, electrons move according to the ballistic mechanism. It has interesting optical, electronic, and mechanical properties and due to its structure, it is the basic building block of carbon-based materials [11]. It has high water permeability, good ion and gas selectivity, and high proton conductivity and these properties led to the application of GO as a solid electrolyte for supercapacitors and batteries. In various electrical devices such as lithium-ion batteries, supercapacitors, and hybrid lithium-ion capacitors, reduced graphene oxide (rGO) is used to fabricate electrode material due to its interesting properties of high conductivity and high surface area [12]. Graphene is used for the construction of a high-performance supercapacitor because it delivers faster electron transfer paths and enhanced stability of the whole hybrid system [13].
1.5 Bismuth Ferrite ( ) as super-capacitor:
BFO is a well-known multiferroic material that has been tested for super-capacitor applications. BFO is present in multiple crystallite phases i.e., and . Hence, during the charging/discharging process it can bear the changes in its phases and show better performance [14]. Bismuth ferrite ( ) is an active material to construct super capacitive electrode because it makes the electrode surface more conducting by providing a more efficient charge transfer pathway. For super capacitance application BFO nano flakes as electrode have been studied with capacitance of 72.2F/g [15] and to enhance capacitance of 350-440F/g Ayan Sarkar et al. have placed the BFO film on . Ferrite materials have low electronic conductivity, resulting in poor super capacitance efficiency. To get better capacitive properties, nanocomposites of BFO with high conducting material are required, because it will provide a large surface area and short conduction parts. For this purpose in the present work, we will synthesize nanocomposite of BFO and reduce graphene oxide to get better results [16].
Graphene is an allotrope of carbon with a single layer of atoms having a 2D hexagonal structure bounded by sp2 orbits as shown in Fig. 1.6. By the mechanical exfoliation process, graphene can be produced from graphite verified by Novoselov et al. in 2004 [17]. Its unique properties including a specific surface area (2630m2 /g), strong Young’s modulus (~1.0TPa), high electron mobility (~15,000 cm2 /V.s), good thermal conductivity (~5000W/mK), and light permeability (~97.7%) attracted increasing attention and due to these feature its demand increasing day by day [18]. Graphene has several applications such as energy storage for supercapacitors, but its zero-gap property limits its use in semiconductors.
Figure 1.7: Structure of Graphene.
There are significant numbers of oxygen in the chemical structure of GO containing functional groups with various planer structures in which sp2 blends into the orbital domain, its 3D structure has both sp2 and sp3 bonds. In traditional structure of GO carbonyl (C=O) and carboxyl groups are present on the edge of GO sheets and hydroxyl and epoxy (C–O–C) groups are mostly present in the basal plane. GO is an insulator due to the presence of these oxygen-containing functional groups and to bring back the original properties of graphene GO must be reduced to remove oxygen-containing functional groups to make reduce graphene oxide [19].
Figure 1.8: Structure of Graphene oxide.
Bismuth Ferrite ( ) is an inorganic compound with rhombohedrally distorted perovskite structure as shown in Fig. 1.8(a) and, because of its multi-ferroic properties at room temperature, a possible candidate for practical applications. The Curie temperature of bismuth ferrite is 1103 K and Néel temperature is 643 K. It is antiferromagnetic material with G-type spin configuration that is; each spin is surrounded by six antiparallel spins on the nearest Fe neighbours along the or directions in its pseudo cubic or rhombohedral structure with lattice parameters =3.965 Å and =89.35° for pseudo-cubic unit cell, = 5.6343 Å and = 59.3480 in the rhombohedral unit cell, Although having a superimposed insufficient cycloid spin structure with a frequency of 620 Å along the room temperature axis, which cancels the macroscopic magnetization and prevents the linear ME effect from being observed[20]. As shown in Fig.1.8 (b), the BFO unit cell can be represented by hexagonal settings having the direction[001] with the lattice parameter = 5.578 Å and = 13.8680 [21] [22].
(a) (b)
Figure 1.9: Schematic diagram of (a) rhombohedral structure of Bismuth Ferrite ( ) [23] (b) hexagonal structure of Bismuth Ferrite ( [24].
In combination with ferromagnetic or antiferromagnetic properties in the same process, multiferroic materials exhibit ferroelectric or anti-ferroelectric properties. As a result, a magnetization transition can be persuaded by an electric field and an external magnetic field can convince electric polarization known as the magnetoelectric effect (ME) effect, and magneto-electrics or seignetto magnets are those materials that display this effect. High current leakage is the main drawback of these materials, so several attempts have been made by doping it in rare earth metals such as lanthanum (La), samarium (Sm), gadolinium (Gd), terbium (Tb) and dysprosium (Dy) etc. to boost its electrical properties.
The decrease of fossil fuels generated an intensive search for alternative natural energy sources. This also triggered research of new storage devices with high storage, power density and long cycle life. Supercapacitors whose storage capacity is much higher than electrolytic capacitors and batteries are important in this regard.
In this project, we aim to develop and understand the parameters that can be tuned to generate and enhance the capacitive response in BFO. Moreover, a composite system with RGO will be prepared as the capacitance is expected to increase with the addition of RGO. The weight percentage of RGO will be varied to find out the best composition which will ensure good performance of designed nano-composite as electrode material for supercapacitor.
CHAPTER: 02
EXPERIMENTAL TECHNIQUES
Chapter # 02
- Experimental Techniques
This chapter covers the synthesis method used in this work and experimental techniques used after the preparation of GO, BFO and BFO-rGO nano-composite to study the structural, morphological, electrochemical and magnetic properties of the given material.
2.1 Synthesis methodology:
The hydrothermal method states a heterogeneous reaction in aqueous media above 100°C and 1bar which is used in material science for the synthesis of the given material. The best examples of hydrothermal reactions are supplied by nature. When Schafhautl observed the formation of quartz microcrystals from silica acid in1845, he gave the first findings of a laboratory-based hydrothermal reaction. Now, in laboratories around the world with perhaps less elegant apparatus, this approach is used.
Figure 2.1: Schematic diagram of the autoclave.
The hydrothermal method is used for single crystal synthesis, which relies on the solubility of minerals under high pressure in hot water. A steel pressure vessel is used for crystal growth called autoclave as shown in Fig.2.1. In comparison to glass and quartz that is inert to both hydrofluoric acid and alkaline media, autoclaves with Teflon inserts use up to 200 ° C and 200 bar as an ideal jar. [25].
In our work, we use hydrothermal method for the synthesis of pure bismuth ferrite and nano-composite of BFO-rGO at 150°C.
Hummers method is used for the preparation of graphene oxide (GO). In this process, 2g of flake graphite and 2g of sodium nitrate (NaNO3) were mixed in the 98 wt. % of H2SO4 with vigorous stirring and then kept this mixture at 50C using ice-bath to obtain a suspension. Now, with continuous stirring of 4hours 12g of potassium permanganate (KMnO4) was added in a suspension mixture. Now, 200ml of deionized water was added to the above mixture with continuous stirring for 2hours and maintained temperature. The temperature was raised to 350C with continuous stirring for 2hours and solution changes its colour from green mixture to brown mixture and then again raised the temperature to 980C with continuous stirring for 10 minutes and finally cools down the mixture at room temperature. Finally, 40ml of hydrogen peroxide (H2O2) was added to above mixture to terminate the reaction and centrifuged with hydrochloric acid (HCl), ethanol and deionized water and then dried at 800C in the microwave oven to get the final product.
2.2.1 X-Ray Diffraction (XRD):
X-Ray Diffraction (XRD) is an analytical technique which is used for the structural conformation of a given material. This technique helps to find out the unknown material by determination of its physical parameters i.e. crystallite size, inter-planar spacing, lattice parameters, lattice strain and crystallographic planes (h, k, l).
In X-Ray Diffraction, the wavelength of X-Rays is of the same magnitude as the size of atoms or ions present in the crystal. The cathode ray tube produces these rays, filters them to produce monochromatic light and then passes them to the sample to be analyzed and detected in the detector. [26]. The detector moves in a circle and record number of X-Rays for each angle of 2θ as shown in Fig. 2.2. The incident rays interact with the sample and produce constructive interference and satisfy Bragg’s law (shown in Fig 2.3 [27]) which is stated as:
nλ=2dsinθ 2.1
Where.
n = positive integer which represent the order of reflection (n= 1, 2, 3…….ꝏ).
λ = wavelength of the incident wave.
D = inter-planar spacing of the crystal.
Θ = scattering angle.
Figure 2.2: Configuration of a Diffractometer system
In X-Ray Diffraction (XRD), the wavelength of the incident wave (λ) is known and angle of incidence (θ) is known then inter-planar spacing (d) of a crystal between lattices planes can be calculated which is used for the identification and characterization purposes. Constructive interference occurs when the incident wave and refracted wave are in phase and the path difference is an integral multiple of wavelength and if the incident and refracted wave are not in phase then X-rays scattered from successive planes and the path difference is an odd integral multiple of the wavelength which result in destructive interference. There are many rational planes of atoms in a structure of given unknown crystal; so, the collection of “reflections” of all the planes can be used to distinctively classify an unknown crystal [28].
In X-Ray Diffraction (XRD) crystallite size can be calculated by using the Debye Scherer equation which is related to peak width which is given as:
2.2
Where λ is the wavelength of X-Ray whose value is 0.15406 nm, K is Scherer constant related to crystallite shape whose value is 0.9, ß is Full-Width Half Maximum (FWHM) in 2θ axis of diffraction profile must be in radian [29]. By analyzing the XRD data, inter-planar spacing can be determined which is further used to determine the lattice parameters and by the help of lattice parameters crystal structure can be obtained. The set of Miller indices uniquely defines a family of crystallographic planes.
In this present work, samples were scanned in 2 range between to 80 with 0.0 step size and 0.5 step second using copper as X-Ray source with 1.54059 wavelength.
Raman spectroscopy is a spectroscopic technique that is used to detect vibrational, rotational and other low-frequency modes. In this technique, the frequency of monochromatic light changes after interaction with the sample and shifted up or down as compared to the original one called the Raman effect. This technique is based on inelastic scattering of monochromatic light which detects vibrations involving a change in polarizability [30].
Figure 2.4: Setup of Raman spectrometer.
The setup of Raman spectrometer consists of a light source usually a laser, focusing mirrors, microscope, Rayleigh filter, Diffracting grating, CCD camera and computer. A monochromatic light falls on focusing mirrors which focus the light and by the help of microscope light shines on the sample and scattered light is collected. Rayleigh filter filters the light except for in-elastically scattered light. Diffracting grating splits the Raman scattered light into a spectrum and CCD camera detect this light and computer analyze detected data. The schematic diagram of a RAMAN spectrometer is shown in Figure 2.4[31].
In Raman spectroscopy, when a photon hits molecules of specimen then they scattered elastically and in-elastically. Elastic scattering occurs when photon diffuse without any changes in energy or frequency called “Rayleigh scattering” (see Fig. 2.5) and inelastic scattering occur when there is a change in energy or frequency called Raman scattering [32]. There are a small number of photons of the order of which are scattered in-elastically indicating a change in frequency and this change in frequency is related to the characteristic vibrations and are fingerprints of a crystal. According to Planck-Einstein, the relation between energy and wavelength is given as:
2.3
2.4
In the above relation, h represents Planck’s constant, c is the speed of light and λ represent the wavelength and this relation shows energy and wavelength are inversely related to each other [33]. When photon strike to the molecules of a specimen than the intensity and wavelength of scattered photon changes as compared to the incident photon which gives chemical and structural information of material to be investigated i.e. bond length, bond angle and low-frequency modes present in a given material. All materials are not considered to be “Raman active”. For a substance to be active in Raman; when an incident beam interacts with specimen atoms, then atoms must begin to oscillate and must travel in different directions that induce a change in the electron cloud, and this creates a separation of charge in atom resulting in a dipole and atom become polarized [34].
Figure 2.5: Energy level diagram of Rayleigh scattering, Raman Stokes and Anti-Stokes.
2.2.3 Scanning Electron Microscopy (SEM):
Scanning electron microscopy is a technique that is used to produce the image of a sample that contains information related to the composition and topography of a sample. The electron beam emitted in this method is used to scan the sample surface and communicate with the atoms present in the sample that produce numerous signals. These signals provide details on the composition and topography of a given sample. Atoms are excited by interaction with an electron beam and secondary electrons are emitted which are detected by using Secondary Electron Detector. The resolution power of SEM is better than 1 nanometer [35].
Figure 2.6: Schematic illustration of SEM.
The working components of SEM include a source of electrons, electromagnetic lenses, electron detector, sample chamber and computer. First of all, electrons are accelerated from electron source and then passed through the arrangement of lenses and aperture which produces a focused beam of electrons which hits the surface of the given sample. Scan coils are positioned above the objective lens, which controls the electron beam location in the sample and scans the beam above the sample surface. By the interaction of electron beam with the sample, various signals are which include secondary electrons, backscattered electrons and characteristics X-ray. Finally, the resulting signals are displayed on a computer screen as shown in Fig. 2.6. In SEM, the maximum resolution depends on different factors like interaction volume of the electron beam with sample and size of electron spot. The resolution of modern SEMs lies between 1 nm to 20 nm [36].
2.2.4 Fourier Transform Infrared Spectroscopy (FTIR):
Fourier Transform Infrared Spectroscopy (FTIR) is a spectroscopic tool that collects high spectral resolution information over a large spectral range at the same time. In this method, a beam of light containing several frequencies falls on the sample at once instead of a monochromatic beam of light, and light absorbed by the sample is measured.
Figure 2.7: Setup of Fourier Transform Infrared Spectroscopy (FTIR)
In this technique, the Michelson interference method was employed which is shown in Fig. 2.7 [37]. A beam of light which is coming from a broadband light source falls on Michelson interferometer in which one mirror moves with the help of a motor. Interferometer blocks and transmits a beam of light periodically for each wavelength due to wave interference. Since various wavelengths are modulated at different rates, a beam coming from an interferometer has a different spectrum. Computers process the raw data into the desired result and turn out to be a common Fourier transform algorithm that converts one domain into an inverse domain[38]. One of the main advantages of using FTIR spectroscopy as compared to other infrared spectroscopy techniques is the ability to measure the spectra with high signals to noise ratios.
In this technique, a graph is plotted between the absorbance of infrared radiation absorbed by the sample material versus wavelength and this infrared absorption bands helps to classify the molecular components and structures [39].
2.2.5 Vibrating Sample Magnetometer (VSM):
The Vibrating Sample Magnetometer (VSM) is a device used to measure the moment of magnetism which detects very small changes in the order of to emu of a sample. With the help of VSM, the magnetic moment of a sample is measured when it is vibrating perpendicular to the magnetizing field and changing magnetic field produces an electric field which is sensed by the pick-up coils.
The schematic diagram of Vibrating Sample Magnetometer is shown in Fig. 2.8 [40]. The sample is vibrated by the loudspeaker assembly perpendicular to the applied field. The voltage is caused by the oscillating magnetic field of the vibrating sample and the magnetic properties of the sample can be detected through this voltage With the assistance of this stationary collection of reference coils, a second voltage is induced by reference sample coils. The resulting voltage amplitude and phase are directly related to each other. The measurements of magnetization are made both as a function of magnetic field and temperature [41].
Figure 2.8: Set-up of Vibrating Sample Magnetometer (VSM).
2.3 Electrochemical measurements:
2.3.1 Cyclic Voltammetry (CV):
Cyclic voltammetry (CV) is an electrochemical technique that is used in molecular species to analyze the mechanism of oxidation and reduction and also to research chemical reactions that involve catalysis that is triggered by electron transfer. Three electrodes are dipped into an electrolyte solution of electrochemical cells called a working electrode, reference electrode and counter electrode The reference electrode is used to calculate the potential added relative to the stable reference response while the current flow between the working and counter electrodes. These electrodes are linked to the potentiostat and using potentiostat software, experimental parameters are selected and a cyclic voltammogram is finally recorded. [42].
Figure 2.9: Electrochemical cell for CV experiment.
CHAPTER: 03
Sample SYNTHESIS
3. Sample Synthesis
This chapter covers the synthesis procedure for the preparation of GO, BFO, and rGO-BFO nano-composite systems.
Chemicals:
| Chemicals
|
Formula | Purity (%) | Molecular Weight (g/mole) | Supplier |
| Sulphuric acid | 95-97% | 98.079g/mole | SIGMA-ALDRICH | |
| Sodium Nitrate | 85% | 97.994g/mole | MERCK | |
| Potassium permanganate | KMn | 99-100.5% | 158.034g/mole | SIGMA-ALDRICH |
| Hydrogen peroxide | 34.5-36.5% | 34.0147g/mole | SIGMA-ALDRICH | |
| Hydrochloric acid | 37% | 36.46g/mole | SIGMA-ALDRICH | |
| Ethanol | 99.8% | 46.07g/mole | BDH Laboratory | |
| Ether | 99.5% | 74.12g/mole | PANREAC SINTESIS | |
| Graphite Powder | G | 99% | 12.01g/mole | SIGMA-ALDRICH |
| Bismuth nitrate pentahydrate | Bi .5 O | 98% | 485.07g/mole | SIGMA-ALDRICH |
| Iron chloride hexahydrate | Fe .6 O | 99% | 270.29g/mole | SIGMA-ALDRICH |
| Acetone | 99.5% | 58.08g/mole | BDH Laboratory | |
| Ammonia | 32% | 17.031g/mole | MERCK | |
| Sodium hydroxide | NaOH | 98-100.5% | 39.997g/mole | SIGMA-ALDRICH |
3.1 Synthesis of GO by using Hummer’s method:
Hummers method is used for the preparation of graphene oxide (GO). In this process, 2g of flake graphite and 2g of sodium nitrate (NaNO3) were mixed in the 98 wt. % of H2SO4 with vigorous stirring and then kept this mixture at 5oC using ice-bath to obtain a suspension. Now, with continuous stirring of 4hours 12g of potassium permanganate (KMnO4) was added in a suspension mixture. Now, 200ml of deionized water was added to the above mixture with continuous stirring for 2 hours and maintained temperature. The temperature was raised to 35oC with continuous stirring for 2 hours and solution changes its colour from green mixture to brown mixture and then again raised the temperature to 98o C with continuous stirring for 10 minutes and finally cools down the mixture at room temperature. Finally, 40ml of hydrogen peroxide (H2O2) was added to the above mixture to terminate the reaction and centrifuged with hydrochloric acid (HCl), ethanol and deionized water and then dried at 80oC in a microwave oven to get the final product.
| 3g of graphite flakes |
| SYNTHESIS OF GRAPHENE OXIDE (GO) |
| 3g of NaNO3 |
+
| Added solution in 90ml of H2SO4 |
| kept at 50C with using icebath |
| Dried at 80 degrees |
| 4h stirring |
| Slowly addition of 12g of KMn |
| Addition of 200ml of DI water with 2h stirring |
| Washing |
| Centrifuged |
| Temperature raised to 980C with 10 minutes stirring |
| Addition of to stop the reaction |
| Temperature raised to 350C with 2h stirring |
Figure 3.1: Graphic representation of the synthesis of Graphene oxide (GO)
3.2 Synthesis of Bismuth Ferrite (BFO):
For the synthesis of BFO we employed hydrothermal method in which we followed the following steps:
In the first phase, we take 2.425 g of Bismuth nitrate pentahydrate (Bi .5 O) and 1.352 g of Iron chloride hexahydrate (Fe .6 O) and were mixed into 50 ml of acetone and sonicated for one hour. In the next phase, the solution was added into 150 ml of deionized water and stirred for one hour. Then, under intense stirring, concentrated ammonia was used as a reducing agent until the solution’s pH value reached 10-11 and then washed with deionized water. In 40 ml of deionized water, red co-precipitate was dispersed. In the next phase, the aqueous solution of 5M NaOH was added under intense stirring into the suspension. At the last stage, the solution was transferred into stainless steel autoclave with Teflon liner and heated at 15 C for 24 hours. Final black powder was separated from the solution and washed with deionized water and dried in the microwave oven at C for 24 hours to obtain the final product.
| SYNTHESIS OF BFO |
| 1.352g of Fe .6 O |
| 50ml acetone |
| 2.425g of Bi .5 O |
+ +
| Stirred for 1hour |
| Add into 150ml DI water |
| SONICATED
(1hour) |
+
| Add ammonia in above solution PH (10-11)
|
| Washing
|
| Drying at 60 degrees in microwave oven
|
| Dispersed into 40ml DI water
|
| Add 5MNaOH in solution (maintained ph 10-11)
|
| Washing
|
| Hydrothermal treatment 150֯֯ degrees (24h)
|
Figure 3.2: Flow chart for the synthesis of Bismuth Ferrite(BFO)
For the synthesis of bismuth ferrite, we employed the hydrothermal method. We prepared two samples of BFO-rGO for different %wt. of GO. First, we prepared a sample with the addition of 1.5% of GO and second with the addition of 3% of GO in a reaction mixture. For the preparation of BFO-rGO we followed the following steps:
In the first phase, 2.425 g of Bismuth nitrate pentahydrate (Bi .5 O) and 1.352 g of Iron chloride hexahydrate (Fe .6 O) were added into 50 ml of acetone and sonicated for one hour. In the next phase, 0.01063g (1.5wt %), and 0.03189g (3wt %) of GO were added in 50 ml of deionized water and sonicated for one hour. In the next phase, both solutions were added into 150 ml of DI (deionized) water and stirred for one hour. Then, under intense stirring, concentrated ammonia was used as a reducing agent until the solution’s pH value reached 10-11 and then washed with deionized water. In 40 ml of deionized water, red co-precipitate was dispersed. In the next phase, the aqueous solution of 5M NaOH was added under intense stirring into the suspension. At the last stage, the solution was transferred into stainless steel autoclave with Teflon liner and heated at C for 24 hours. The product was separated from the solution and washed with deionized water and then dried in the microwave oven at C for 24 hours.
| 2.425g of Bi .5 O
|
| 50ml acetone
|
| 50ml DI water
|
| Synthesis OF BFO-rGO |
| 0.3189g of GO
|
| 1.352g of Fe .6 O
|
| Sonicated both samples for 1hour |
SAMPLE (A) SAMPLE (B)
| Add both solutions into 150ml DI water and stirred for 1 hour |
| Addition of with vigorous stirring (Maintain ph 10-11) |
| Washing |
| Finally, dried at 70 degrees in microwave oven (24h) |
| Hydrothermal treatment at 150 degrees (24h) |
| Add 5M NaOH aqueous solution in given solution with vigorous stirring |
| Add solution into 40ml DI water |
| Washing with DI water in centrifuge machine |
Figure 3.3: Flow chart for the synthesis of BFO-rGO nanocomposite
Chapter#04
Results and Discussion
Chapter # 04
4. Results and Discussion
This chapter deals with the structural, electronic, and magnetic properties of BFO, rGO and BFO-rGO nano-composite systems. The discussion of our experimental work is divided into the following parts.
- Structural analysis by using X-ray diffraction (XRD).
- Analysis of vibrational modes by using RAMAN spectroscopy.
- Study of magnetic properties through Vibrating Sample Magnetometer (VSM).
4.1.1 Comparison of XRD patterns of Graphite, Graphene oxide (GO) and Reduce Graphene oxide (rGO):
X-Ray Diffraction patterns of Graphite, Graphene oxide (GO), Reduce Graphene oxide (rGO) taken at room temperature are shown in Fig.4.1. The diffraction peaks of Graphite, GO and rGO has been observed at 26.680, 10.010 and 26.870 with
For Graphite, one sharp diffraction peak has been observed at 2θ = 26.680 corresponding to (002) plane with a d-spacing of 0.339 nm which is consistent with the literature [26]. For GO, the peak is shifted to 2θ = 10.010 corresponding to (001) orientation with a d-spacing of 0.882 nm which confirms the oxidation of graphite into GO and is consistent with the literature [43]. For rGO, the diffraction peak is shifted to 26.870 which corresponds to (002) plane and has a d-spacing of 0.345 nm. The shift of diffraction peak to higher angle is attributed to the removal of oxygen-containing functional groups. The reduction of interlayer distance confirmed that GO is successfully reduced to rGO and is consistent with the literature [44]. All observed d (interlayer distance) values for Graphite (0.339 nm), GO (0.882 nm), and rGO (0.345 nm) are consistent with the literature. The d values were calculated using Bragg’s law given by equation 4.1
2dsinθ = nλ (4.1)
Where d is the interlayer distance, n is the order of diffraction and λ is the wavelength of X-ray source which is equal to 0.15406 nm for copper Ka source.
Figure 4.1: XRD of Graphite, GO, rGO samples measured at room temperature.
4.1.2 XRD of Pure BiFeO3 (BFO):
XRD pattern of pure BFO obtained at room temperature in 2θ range between 100 to 700 and is shown in Fig.4.2.The diffraction peaks of BFO has been observed at angle of 22.410, 31.780, 32.080, 45.730, 51.360, 51.760, 55.180, 56.730, 65.780, 67.090 corresponding to (012), (104), (110), (006), (202), (024), (116), (122), (018), (214), (208) and (220) basal planes which are in agreement with literature [45]. The observed diffraction pattern confirms rhombohedrally distorted perovskite structure having the space group of R3c. However, along with the diffraction peaks of BFO, very low-intensity additional peaks have been observed which correspond to the Bi deficient Bi2Fe4O9 impurity phase. This phase has been commonly observed in the case of BFO [46, 47]. The diffraction peaks for BFO are very sharp which indicates the crystalline nature of the BFO sample [48].
Figure 4.2: XRD of Pure BFO sample obtained at room temperature.
BFO unit cell can be described by hexagonal setting as well so we calculated lattice parameters using the relation given in equation 4.2,
(4.2)
Where d is inter-planer spacing which is calculated by using Bragg’s law, a and c are lattice parameters and h, k, l are miller indices. The calculated lattice parameters of BFO are a = b = 5.575 Å and c = 13.843Å and volume is V = 66.835 Å3.These values are consistent with the literature [49]. Crystallite size is calculated by using the Debye–Scherrer equation given by equation 4.3,
(4.3)
Where D is crystallite size, k is Scherrer constant equal to 0.9, β is full-width half maxima (FWHM) and λ is the wavelength of the X-ray source.
The crystallite size calculated for pure BFO sample is given in Table 4.1. The size was calculated using various planes and then the average crystallite size was obtained which is 37.08 nm.
Table 4.1: Crystallite size calculations for pure BFO sample.
| Miller indices | 2Theta | Theta | Theta | FWHM | FWHM | D |
| (degrees) | (degrees) | (radian) | (degrees) | (radian) | (nm) | |
| (012) | 22.41985 | 11.20993 | 0.19564 | 0.22523 | 0.00393 | 35.95894 |
| (104) | 31.78699 | 15.8935 | 0.27739 | 0.23428 | 0.00409 | 35.25813 |
| (110) | 32.08878 | 16.04439 | 0.28002 | 0.19715 | 0.00344 | 41.93001 |
| (202) | 39.52575 | 19.76288 | 0.34492 | 0.23988 | 0.00419 | 35.19139 |
4.1.3 XRD of BFO-rGO nanocomposite:
The X-Ray Diffraction pattern of BFO-rGO nanocomposite obtained at room temperature in 2θ range between 100 to 700 are shown in Fig.4.1. The diffraction peaks of BFO has been observed at angles of 22.410, 31.780, 32.080, 45.730, 51.360, 51.760, 55.180, 56.730, 65.780 and 67.090 corresponding to (012), (104), (110), (006), (202), (024), (116), (122), (018), (214), (208) and (220) basal planes which are consistent with the literature [45] which are characteristics of rhombohedrally distorted perovskite structure belonging to R3c space group. However, along with the diffraction pattern of pure BFO, some additional low-intensity peaks have been observed. The diffraction peak observed at 26.870 belong to rGO phase which confirms the reduction of GO to rGO during hydrothermal treatment. As can be confirmed from Fig 4.1, that diffraction peak for GO (001) should be present at 10.010. This peak has been shifted to 26.870 confirming the successful reduction of GO which is in great agreement with the literature [44]. Moreover, a minute amount of Bi2Fe4O9 impurity phase was also observed. This phase is very difficult to avoid and has been reported by several authors [46, 47].
Figure 4.3: XRD of BFO-rGO nanocomposite measured at room temperature.
4.2.1 Raman Spectra of GO & rGO:
The structure of GO and rGO samples and BFO-rGO nanocomposites was further studied by Raman spectroscopy. Raman spectra of Graphene oxide(GO) and reduced graphene oxide(rGO) is shown in Fig. 4.4. Typically, two bands exist in graphene or its derivatives which are assigned as G and D bands. The G band arises from the first-order scattering of E2g phonons due to sp2 hybridized carbon atoms whereas the D band arises due to the structural imperfections introduced in graphitic layers by oxygen-containing functional groups. These functional groups attach themselves on carbon basal plane and are sp3 hybridized. In both samples GO and rGO, G band is located at 1606 cm-1 and 1618 cm-1 whereby the D band is located at 1381 cm-1 and 1357cm-1, respectively. The ratio of intensities ID/IG is 0.96 and 1.22 for GO and rGO, respectively. The increase in ID/IG ratio is commonly attributed to the successful reduction of GO into rGO [50]. Huge quantity of various smaller size sp2 domains (graphene sheets) is created in the reduction of GO into rGO due to which the overall sp2 domain average size is decreased. These smaller sized sp2 domains may lead to large structural defects which eventually cause an increase in the intensity of the D band [50]. Removal of oxygen-containing functional groups from graphene sheets leads to the increased graphene edges which could also be responsible for the increase in D band intensity [51]. The sp2 and sp3 domain in case of GO is shown in Fig. 4.5. The differences in case of GO and rGO are shown in Fig. 4.6, which shows that more sp2 domains are obtained with smaller domain size in case of rGO but with a higher density of structural defects. This will lead to an increase in the intensity of the D band. Moreover, a 2D band (around 2700 cm-1) which is also known as secondary D band has been observed in the case of rGO sample. In single-layer graphene, this band normally has high intensity, but in multi-layer graphene, it widens and decreases in intensity. Typically, as you can see from graphite, there is not much difference if it is more than five layers.
4.2.2 Raman analysis of Pure BFO:
Raman spectroscopy measurement was performed to study various Raman modes present in single-phase and composite systems. The deconvoluted Raman spectra of pure BFO in the range of 100 cm-1to 1500 cm-1 obtained at room temperature is shown in Fig.4.7. BFO has 10 atoms in the unit cell of perovskite structure which according to group theory can produce 18 phonon modes (4A-1+5A-2+9E). Out of which 13 modes (Г = 4A1 + 9E) are Raman activation modes [52] which are given in Table 4.2. For pure BFO, A1 and E modes are Raman active modes and A2 mode is not active in Raman. Raman active modes are found at 145cm-1, 173cm-1, 228cm-1, 429cm-1 which are assigned as A11, A12, A13, A14 modes. The modes obtained at 268cm-1, 281cm-1, 345cm-1, 375cm-1, 417cm-1, 466cm-1, 528cm-1, 594cm-1 are assigned as E modes. All these modes are consistent with the literature[53]. In BFO lattice Bi atoms contribute to the low-frequency mode below 167cm-1 while Fe atoms contribute to high-frequency mode above 262cm-1. The modes A11, A12, A13, E1 and E2 are associated with the covalent bond Bi-O, while the modes E-3 and E-4 are associated with the covalent bond Fe-O. The expansion and change of modes show that vacancies of oxygen are generated in the system that will lead to distortion of pure BFO in lattice.
4.2.3 Raman analysis of nano-composite BFO-rGO:
Raman spectra of BFO-rGO (3%) nano- measured in the range between 100 cm-1 to 1500 cm-1 is shown in Fig.4.8. Raman active modes found at 145cm-1, 173cm-1, 228cm-1, 429cm-1 are assigned as A11, A12, A13, A14 modes and the modes observed around 268cm-1, 281cm-1, 345cm-1, 375cm-1, 417cm-1, 466cm-1, 528cm-1, 594cm-1 are assigned as E modes which are consistent with literature [53]. The two main peaks were found at 1632cm-1and 1368cm-1 are assigned as D and G bands corresponding to rGO phase. and agree with the literature [49]. These peaks exist due to the scattering of E2g phonons in the ordered graphene sp2 -bonded carbon (G peak) and characteristic signals of defects and disorder in the hexagonal graphite layer (D peak). The Raman spectrum of nano-composite BFO-rGO has 13 active vibrational peaks (Г=4A1+9E) based on literature[52, 54] given in table 4.1 which shows there is no structural change in the BFO powder after hydrothermal treatment [55]. The schematic representation of A and E modes is given in Fig. 4.9.
Table 4.2: Raman modes of pure BFO and nano-composite BFO-rGO samples.
| Raman modes | Raman modes of BFO(our study) | Raman modes of BFO-rGO
(our study) |
Raman modes (reference)[53] |
| A1-1 | 145cm-1 | 145cm-1 | (147,131)cm-1 |
| A1-2 | 173cm-1 | 173cm-1 | (176,168)cm-1 |
| A1-3 | 228cm-1 | 227cm-1 | (227,212)cm-1 |
| A4-4 | 429cm-1 | 429cm-1 | (490,488)cm-1 |
| E | 268cm-1 | 268cm-1 | (265,272)cm-1 |
| E | 281cm-1 | 281cm-1 | (279) cm-1 |
| E | 345cm-1 | 345cm-1 | (351,345)cm-1 |
| E | 375cm-1 | 375cm-1 | (375)cm-1 |
| E | 417cm-1 | 417cm-1 | (437,416)cm-1 |
| E | 466cm-1 | 466cm-1 | (473,488)cm-1 |
| E | 528cm-1 | 528cm-1 | (525,532)cm-1 |
| E | 594cm-1 | 594cm-1 | (577,590)cm-1 |
4.2.4 Comparison of carbon-related peaks of BFO-rGO (3%) with pure GO:
Raman analysis of BFO-rGO nano-composite and GO is shown in Fig 4.10. In Raman spectra of GO and BFO-rGO nano-composite, two bands exist which are called D-band and G-band. For GO, peaks of G-band and D-band were found at 1606cm-1 and 1381cm-1 as already discussed. For BFO-rGO(3%) sample, peaks of G-band and D-band were found at 1632cm-1and 1368cm-1, respectively which are consistent with the literature [54]. As already discussed, the G band represent C-C stretching which arises from the first-order scattering of E2g phonons due to sp2 hybridized carbon atoms and the D band represent defects which arise due to the structural imperfections introduced in graphitic layers by oxygen-containing functional groups. It can be seen in the figure that the Raman peak of D-band is shifted toward lower values while the Raman peak of G-band is shifted toward higher values for BFO-rGO nanocomposite compared with GO. This shift can be attributed to defects introduced in the lattice after reduction and removal of oxygen-containing functional groups which cause displacement of atoms. The FWHM of D and G bands in BFO-rGO is significantly higher than D and G bands observed for GO. This increase can be attributed to the increase in structural defects after reduction [51]. The ID/IG ratio for pure GO is ~0.9 and for BFO-rGO is ~1.01 which also confirms an increase in structural defects and is consistent with the literature [55]. Another band called 2D band (Secondary D-band) are also observed in both samples. It is known that the intensity of the 2D band is higher in single-layer graphene as compared to multilayer graphene.
4.3 Magnetic measurements of pure BFO and BFO-rGO nano-composite:
Magnetic measurements of synthesized BFO and BFO-rGO (3%) nano-composite measured at room temperature up to a magnetic field of 30,000 Oe (3 Tesla) are shown in Fig.4.11. The magnetic measurements are given in Table 4.3 [56, 57].
| Material | Saturation magnetization (emu/g) | Coercivity (Hc)
(Oe) |
Remanent magnetization (emu/g) |
| Pure BFO | 4.24 | 160.58 | 0.0346 |
| 3% BFO-rGO | 3.79 | 157.23 | 0.0319 |
Table 4.3: Magnetic measurements of Pure BFO and BFO-rGO samples.
Both samples exhibited weak ferromagnetic behaviour as observed from the hysteresis.
The zoomed-in view of M-H loops is also shown in Fig. 4.11(b) from which a clear opening of the loop can be observed. The values of magnetic parameters such as saturation magnetization (MS), remanent magnetization (Mr) and magnetic coercivity are given in table 4.3. The value of Ms for pure BFO sample is 4.24 emu/g where the value for BFO-Rgo nanocomposite is 3.79 emu/g. A slight decrease in magnetization for nanocomposite has been observed which is attributed to the non-magnetic nature of rGO component.
(a) (b)
Figure 4.11: (a) Magnetic hysteresis loop of Pure BFO and BFO-rGO(3%) nano-composite (b) Rescaled axis shows the respective coercivity and Remanent magnetization of Pure BFO and BFO-rGO(3%) nano-composite.
Magnetic properties of BFO were earlier studied by Sosnowska et al. [58]. He confirmed that BFO has G-type antiferromagnetic structure and has a Néel temperature of TN ~ 643K. In G-type configuration each Fe3+ ion is surrounded by six of the nearest Fe ions with opposite spins.
It has been detected that tilting of the FeO6 octahedra reduces the Fe−O−Fe angle from 180°, reducing the overlap of Fe d and O 2p orbitals and results in the decrease of Fe−O−Fe angle to 154−156°. If the Fe−O−Fe angle was 180° one would assume collinear antiferromagnetism (Fig. 4.12(a). Dzyaloshinskii-Moriya (DM) interaction between Fe atoms resulted in slight canting of magnetic moments which resulted in weak magnetization in the material. (Fig 4.12(a)) [59-61] However, G-type structure is superimposed by a spin spiral (spiral direction along [110] and a spin rotation plane of (110)) which has a length of 62nm which resulted in the cancellation of net moment previously originated by canting magnetic moments. (Fig. 4.12(b)) [58]. So the magnetic moment in BFO can only be observed, once this spiral spin structure is suppressed. The spiral spin structure has been reported to be suppressed in various ways, such as the application of high magnetic fields (about 18 T)[62] due to epitaxial constraints in finite-size effects in thin films [63] in nanosized BFO and the implementation of structural changes by appropriate cationic substitution [64, 65].
The observed magnetic behaviour in our case is because of the finite-size effects. The particle sizes in our case our around 30 nm which is less than the length of the spiral spin structure which resulted in net magnetization.
Figure 4.12: (a) Weak ferromagnetism induced by canted spins sublattices. (b) Schematic diagram of the cycloid spiral spin structure of BFO.
5. Conclusion
The objective of this study was to fabricate a super-capacitive material and understand the parameters that can be tuned to generate and enhance the capacitive response. In this project, a nano-composite system of BiFeO3 (BFO) and reduced graphene oxide (rGO) was synthesized using a facile hydrothermal method and their structural, vibrational, magnetic, and electrochemical impedance measurements have been studied. Graphene is a suitable candidate for such applications due to large surface to volume ratio, high conductivity, and high capacitance. On the other hand, BFO is a multiferroic material which has very high polarization and hence capacitance. Thus, the (1-x)BFO-xRGO (0 ≤ x ≤ 0.03) nano-composite system can be an attractive candidate for high-performance supercapacitor electrode. The ratio of the two components has been optimized to obtain the desired properties.
Structure and phase purity were investigated by X-ray powder diffraction (XRD) and Raman spectroscopy whereas magnetic properties were analyzed by a vibrating sample magnetometer (VSM). XRD pattern of single-phase BFO nanoparticles and the BFO-rGO composite sample showed characteristic diffraction peaks of BFO which confirmed the successful formation of the perovskite structure. The GO peak at ~10° vanished and a small peak at around ~26° was observed in the BFO-rGO composite which showed the successful reduction of GO into rGO during the hydrothermal reaction. The reduction of GO into rGO in one-step hydrothermal reaction hypothesis was further confirmed by Raman analysis where D and G band were observed with strong intensities. The ratio of intensities of D and G bands ID/IG = 0.9 observed in GO increased to ID/IG = 1.01 for the BFO-rGO sample which confirms the successful reduction of GO to rGO. The intensity of the D band increases significantly due to the exclusion of sp3 bonded oxygen functionalities. All the expected vibrational modes have been observed for both BFO and nanocomposites. The saturation magnetization of BFO is 4.24 emu/g and for BFO-rGO nanocomposite is 3.79 emu/g. This small decrease is attributed to the non-magnetic rGO phase in BFO-rGO nanocomposite. The electrochemical measurement results are still awaited due to delays caused by COVID-19 issues. We are expecting enhanced electrochemical properties for the case of nanocomposite systems.